Learning Outcomes
On completion of the course, the student should be able to:
1. identify and deduce the physical quantities and their units;
2. differentiate between vectors and scalars;
3. describe and evaluate motion of systems on the basis of the fundamental laws of mechanics;
4. apply Newton’s laws to describe and solve simple problems of motion;
5. evaluate work, energy, velocity, momentum, acceleration, and torque of moving or rotating objects;
6. explain and apply the principles of conservation of energy, linear and angular momentum;
7. describe the laws governing motion under gravity; and
8. explain motion under gravity and quantitatively determine behaviour of objects moving under gravity.
Course Contents
space and time. units and dimension. vectors and scalars. differentiation of vectors: displacement, velocity and acceleration. Kinematics. Newton laws of motion (Inertial frames, Impulse, force and action at a distance, momentum conservation). relative motion. Application of Newtonian mechanics. equations of motion. conservation principles in physics, conservative forces, conservation of linear momentum, Kinetic energy and work, Potential energy, System of particles, Centre of mass. Rotational motion. torque, vector product, moment, rotation of coordinate axes and angular momentum, polar coordinates. conservation of angular momentum; Circular motion. Moments of inertia, gyroscopes and precession. gravitation: Newton’s Law of Gravitation, Kepler’s Laws of Planetary Motion, Gravitational Potential Energy, Escape velocity, Satellites motion and orbits.
PART A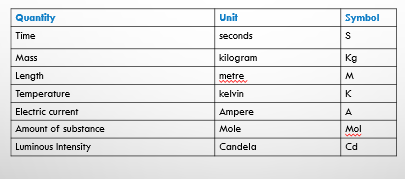
Derived quantities are those quantities that derived from the fundamental quantities. Their units are known as derived units. Examples are:
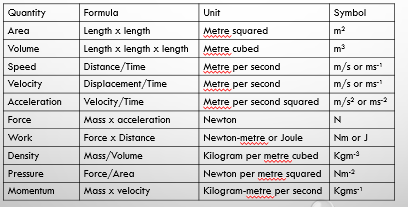

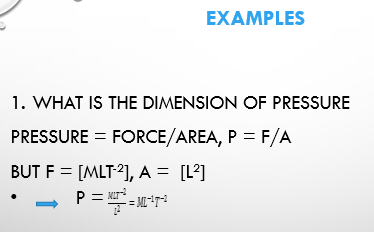
Uses of dimensions:

SCALAR AND VECTOR QUANTITIES

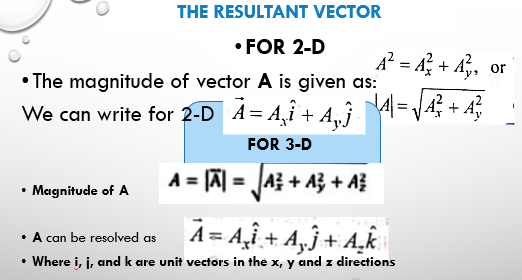
Vector direction: In 2-D, the angle that vector A makes with the positive x-axis is given as:
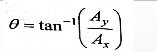
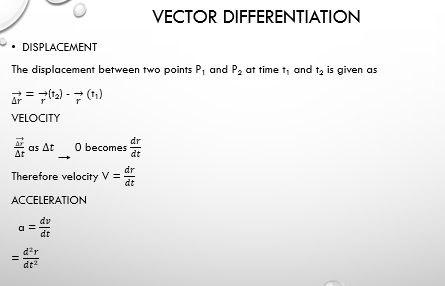
Unit vector: A unit vector is a vector having a unit magnitude. It is used to describe the direction of the vector. It is given as:


VECTOR ADDITION: The process in which to or more vectors are added to get a single vector is called vector addition. This single vector is known as resultant vector. It has the same effect as the other vectors combined together.

Given: A = 2i + 3j + 2k , B = i + 2j + 4k.
A +B = (2i + 3j + 2k )+ (i + 2j + 4k)
Adding i to i, j to j and k to k
= 2i +i + 3j + 2j +2k + 4k
= 3i + 5j + 6k
N.B: Addition of vector gives a vector
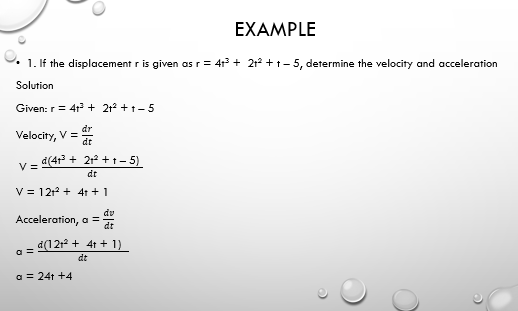
- Lecturer : Agatha Oyiogu
